Professor Eilon Solan is the Nathan and Lily Silver Chair in Stochastic Models at the School of Mathematical Sciences at Tel-Aviv University in Israel. He is also the director of the Good To Know project.

Eilon recently visited the department earlier this year to collaborate with Dr Ashkenazi-Golan. During this visit, we caught up with Eilon to discuss his life before academia, his research, and his love of history.

Eilon Solan

Hi Eilon, thank you for joining us. Could you start by telling us about your life before academia?

I grew up in Israel, in a town next to Tel Aviv. As a teenager, I developed a love for computer science and my first computer was the Sinclair ZX, which was very small and cheap. You would connect it to the TV, which would become the screen, and all you could do was program using the BASIC program language. Even then, the memory was so small that you could not do much more than basic programming. However, it was enough to make me want to enrol in university and develop my understanding. The aspiration to go to university was also unusual as most people in my neighbourhood did not enter further education. Indeed, Israel has mandatory national service at eighteen, so, it would be rare for people to go to university straight after school. However, there was a scheme in which you could defer your army service until after university, but then you would serve additional years, so I chose to do this. I had also always loved maths as well as computer science, so I chose to study computer science with mathematics as my minor.

What inspired you to do a mathematics PhD in game theory?

After one year of undergraduate studies, I realised that I found mathematics more interesting than computer science, so I decided to major in mathematics. However, it wasn’t until the third year that I realised I wanted to pursue a PhD in game theory. I was also fortunate enough to be taught by a great game theorist named Abraham Neyman, which also helped me make my decision.

Every week, he would enter the classroom, put his sunglasses and his keys on his desk, and start teaching without notes. He had everything perfectly memorised, and he taught this very mathematically demanding content clearly and understandably. I enjoyed his course, and I admired him. It was then that I decided to do my PhD with Prof. Neyman. However, I first had to serve in the army for six years, but I was able to do my master’s during this period, and even started my PhD. Two and a half years after I finished my army service, I completed my PhD and continued into academia.

Can you tell us a bit about your research?

In the game theory that you might first learn, a game is a payoff matrix, which tells you the payoffs of the players in the game if they take certain actions. We assume that all players aim to maximise their payoffs, and we define an equilibrium to be a vector of actions, one for each player, such that no player can profitably deviate from their action, even if they know the actions of the other players.

My research is in dynamic games. These are games where there is repeated interaction between players, and I am firstly interested in how players can best play these games, which involves finding and analysing equilibria. But I am also interested in games where some players may have more or less information than others, and the question is how they can best use their private information in these games. Very often, I will study various economic models, but it is not these economic applications that motivate me but the pure mathematically challenging questions.

John Nash

Can you tell us about an open problem in game theory that you have worked on?

Yes, the open problem I was given as a PhD student is still open. In the 1950s, John Nash proved that if a game is only played once, then an equilibrium always exists when players are allowed to randomise their choice of action. Now suppose that we do not play only once but repeatedly play against each other. And, each time we play, our payoffs are given by a different matrix, and the outcome of each game decides which matrix we will play next. Suppose that we do this without end. The question is whether an equilibrium exists.

For two players, this question was solved by Nicolas Vieille in 2000, but we do not know for three players, and this is an area of ongoing research, including here at LSE. These types of games are called Stochastic Games.

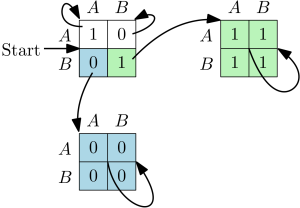
An example of a 2-player stochastic game called the “Big Match”. We start with the 2-player zero-sum game in the top-left, where the entries in the matrix show the payoffs to the row player. The column player and the row player each choose between ‘A’ and ‘B’, and row player wins in each round that the two players pick the same option (otherwise the column player wins the round). As long as the row player chooses ‘A’, the game keeps repeating; but if the row player chooses ‘B’ in round t, the game moves to a new payoff matrix (as shown by the arrows); the winner in round t then wins all future rounds.

That is very interesting and somewhat surprising! What do you enjoy the most and least about research?

What I enjoy most about research is the moment you find the solution you have been working towards. Or it might be when you succeed in combining tools from different areas and can apply them to solve a question that does not initially seem related. This is very satisfying as well.

What I enjoy the least is when I have spent the whole summer working on a solution to a problem, only to realise that everything I had thought is wrong!

What advice would you have for an undergraduate student who is thinking of doing an MSc or a PhD?

Do whatever you like the most. Don’t listen to those who tell you to study the subjects that will earn you more money. This is your life, and you should enjoy it as much as you can.

Suppose that you weren’t a mathematician. What else would you be?

Maybe I would be a historian. I would love to spend my days studying Antiquity. Whenever I visit the British Museum, I am fascinated by Assyria, Babylon, and Ancient Greece. I would give almost anything to walk the streets of Ancient Athens.

Thank you, Eilon. It has been a pleasure!